QUESTION BANK CLASS 6 BASIC GEOMETRICAL IDEAS
- How many lines can pass through two given points?
a) 2 b) 0 c) 1 d) infinitely
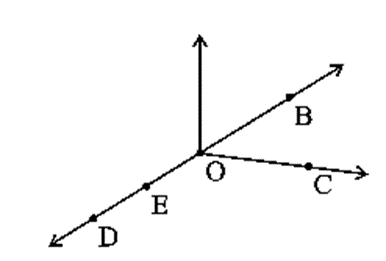
- In the given diagram, name the point in the exterior of ∠EOF
- In the given diagram, name the point in the interior of ∠DOE
a) C b) E c) B d) A
- Name the line given in all possible (twelve) ways, choosing only two letters at a time from the four given. (3M)
- Use the figure to name:
(a) Five points
(c) Four rays
(d) Five line segments
- Name the angles in the given figure
EXERCISE 4.1
1. Use the figure to name :
(a) Five points
(b) A line
(c) Four rays
(d) Five line segments
2. Name the line given in all possible (twelve) ways, choosing only two letters at a
time from the four given.
3. Use the figure to name :
(a) Line containing point E.
(b) Line passing through A.
(c) Line on which O lies
(d) Two pairs of intersecting lines.
4. How many lines can pass through (a) one given point? (b) two given points?
5. Draw a rough figure and label suitably in each of the following cases:
6. Consider the following figure of line MN . Say whether following statements are true
or false in context of the given figure.
EXERCISE 4.2
EXERCISE 4.2
1. Classify the following curves as
(i) Open or
(ii) Closed.
2. Draw rough diagrams to illustrate the following :
(a) Open curve
(b) Closed curve.
3. Draw any polygon and shade its interior.
4. Consider the given figure and answer the questions :
(a) Is it a curve?
(b) Is it closed?
5. Illustrate, if possible, each one of the following with a rough diagram:
(a) A closed curve that is not a polygon.
(b) An open curve made up entirely of line segments.
(c) A polygon with two sides.
EXERCISE 4.3
1. Name the angles in the given figure.
2. In the given diagram, name the point(s)
(a) In the interior of ∠DOE
(b) In the exterior of ∠EOF
(c) On ∠EOF
3. Draw rough diagrams of two angles such that they
have
(a) One point in common.
(b) Two points in common.
(c) Three points in common.
(d) Four points in common.
(e) One ray in common.
EXERCISE 4.4
1. Draw a rough sketch of a triangle ABC. Mark a point P in its interior and a point Q in
its exterior. Is the point A in its exterior or in its interior?
2. (a) Identify three triangles in the figure.
(b) Write the names of seven angles.
(c) Write the names of six line segments.
(d) Which two triangles have ∠B as common?
EXERCISE 4.5
1. Draw a rough sketch of a
quadrilateral PQRS.
Draw its diagonals.
Name them.
Is the meeting point of the diagonals in the interior or
exterior of the quadrilateral?
2. Draw a rough sketch of a quadrilateral KLMN.
State,
(a) two pairs of opposite sides,
(b) two pairs of opposite angles,
(c) two pairs of adjacent sides,
(d) two pairs of adjacent angles.
3. Investigate :
Use strips and fasteners to make a triangle and a quadrilateral.
Try to push inward at any one vertex of the triangle.
Do the same to the quadrilateral.
Is the triangle distorted?
Is the quadrilateral distorted?
Is the triangle rigid?
Why is it that structures like electric towers make use of triangular shapes and not
quadrilaterals?
EXERCISE 4.6
1. From the figure, identify :
(a) the centre of circle
(b) three radii
(c) a diameter
(d) a chord
(e) two points in the interior
(f) a point in the exterior
(g) a sector
(h) a segment
2. (a) Is every diameter of a circle also a chord?
(b) Is every chord of a circle also a diameter?
3. Draw any circle and mark
(a) its centre
(b) a radius
(c) a diameter
(d) a sector
(e) a segment
(f) a point in its interior
(g) a point in its exterior
(h) an arc
4. Say true or false :
(a) Two diameters of a circle will necessarily intersect.
(b) The centre of a circle is always in its interior.
POINTS TO REMEMBER
1. A point determines a location. It is usually denoted by a capital letter.
2. A line segment corresponds to the shortest distance between two points. The line
segment joining points A and B is denoted by AB.
3. A line is obtained when a line segment like AB is extended on both sides indefinitely;
it is denoted by AB
s ruu
or sometimes by a single small letter like l.
4. Two distinct lines meeting at a point are called intersecting lines.
5. Two lines in a plane are said to be parallel if they do not meet.
6. A ray is a portion of line starting at a point and going in one direction endlessly.
7. Any drawing (straight or non-straight) done without lifting the pencil may be
called a curve. In this sense, a line is also a curve.
8. A simple curve is one that does not cross itself.
9. A curve is said to be closed if its ends are joined; otherwise it is said to be open.
10. A polygon is a simple closed curve made up of line segments. Here,
(i) The line segments are the sides of the polygon.
(ii) Any two sides with a common end point are adjacent sides.
(iii) The meeting point of a pair of sides is called a vertex.
(iv) The end points of the same side are adjacent vertices.
(v) The join of any two non-adjacent vertices is a diagonal.
11. An angle is made up of two rays starting from a common starting/initial point.
Two rays OA
u ruu
and OB
u ruu
make ∠AOB(or also called ∠BOA ).
An angle leads to three divisions of a region:
On the angle, the interior of the angle and the exterior of the angle.
12. A triangle is a three-sided polygon.
13. A quadrilateral is a four-sided polygon. (It should be named cyclically).
In any quadrilateral ABCD, AB & DC and AD & BC are pairs of opposite
sides.
∠A & ∠C and ∠B & ∠D are pairs of opposite angles.
∠A is adjacent
to ∠B & ∠D ; similar relations exist for other three angles.
14. A circle is the path of a point moving at the same distance from a fixed point.
The fixed point is the centre,
the fixed distance is the radius and the distance
around the circle is the circumference.
A chord of a circle is a line segment joining any two points on the circle.
A diameter is a chord passing through the centre of the circle.
A sector is the region in the interior of a circle enclosed by an arc on one side
and a pair of radii on the other two sides.
A segment of a circle is a region in the interior of the circle enclosed by an arc
and a chord.
The diameter of a circle divides it into two semi-circles
No comments:
Post a Comment