CLASS 8 MATHS 9 Algebraic Expressions QUESTIONS

 9 Algebraic Expressions And Identities

9.1 What Are Expressions

 9.2 Terms Factors And Coefficients

9.3 Monomials Binomials And Polynomials

9.4 Like And Unlike Terms

9.5 Addition And Subtraction Of Algebraic Expressions 

 9.6 Multiplication Of Algebraic Expressions

9.7 Multiplying A Monomial Monomial

9.7.1 Multiplying Two Monomials

9.7.2 Multiplying Three Or More Monomials

9.8 Multiplying A Monomial By A Polynomial

9.8.1 Multiplying Monomial By A Binomial

9.8.2 Multiplying Monomial By A Trinomial

9.9 Multiplying A Polynomial By A Polynomial

9.9.1 Multiplying A Binomial By Binomial

9.9.2 Multiplying Binomial By Trinomial

9.10 What Is An Identity

9.11 Standard Identities

9.12 Applying Identities10 Visualising Solid Shapes

Example 1: Add: 7xy + 5yz – 3zx, 4yz + 9zx – 4y , –3xz + 5x – 2xy.

Solution:7xy + 5yz –3zx + 4yz + 9zx – 4y + –2xy – 3zx + 5x =

5xy + 9yz +3zx + 5x – 4y

Example 2: Subtract 5x2 – 4y2 + 6y – 3 from 7x2 – 4xy + 8y2 + 5x – 3y. Solution: 

7x2 – 4xy + 8y2 + 5x – 3y 

5x2 – 4y2 + 6y – 3

(–) (+) (–) (+) 

2x2 – 4xy + 12y2 + 5x – 9y + 3

EXERCISE 9.1 

1. Identify he terms, their coefficients for each of the following expressions. 

(i) 5xyz2 – 3zy

(ii) 1 + x + x2

(iii) 4x2y2 – 4x2y2z2 + z2 

(iv) 3 – pq + qr – rp

(v) x/2 + y/2 − xy

(vi) 0.3a – 0.6ab + 0.5b 

2. Classify the following polynomials as monomials, binomials, trinomials. Which polynomials do not fit in any of these three categories? 

x + y, 1000, x + x2 + x3 + x4, 7 + y + 5x, 2y – 3y2, 2y – 3y2 + 4y3, 5x – 4y + 3xy, 4z – 15z2, ab + bc + cd + da, pqr, p2q + pq2, 2p + 2q 

3. Add the following. 

(i) ab – bc, bc – ca, ca – ab

(ii) a – b + ab, b – c + bc, c – a + ac

(iii) 2p2q2 – 3pq + 4, 5 + 7pq – 3p2q2

(iv) l2 + m2, m2 + n2, n2 + l2, 2lm + 2mn + 2nl 

4. (a) Subtract 4a – 7ab + 3b + 12 from 12a – 9ab + 5b – 3 

(b) Subtract 3xy + 5yz – 7zx from 5xy – 2yz – 2zx + 10xyz 

(c) Subtract 4p2q – 3pq + 5pq2 – 8p + 7q – 10 from 18 – 3p – 11q + 5pq – pq2 + 5p2q

Example 3: Complete the table for area of a rectangle with given length and breadth. 

Solution:

length breadth area 

3x 5y 3x × 5y = 15xy 

9y 4y2.............. 

4ab 5bc .............. 

2l2m 3lm2.............. 

Example 4: Find the volume of each rectangular box with given length, breadth and height.

length breadth height 

(i) 2ax 3by 5cz 

(ii) m2n n2p p2m 

(iii) 2q 4q2 8q3 

Solution: Volume = length × breadth × height 

Hence, for (i) volume = (2ax) × (3by) × (5cz) = 2 × 3 × 5 × (ax) × (by) × (cz) = 30abcxyz 

 for (ii) volume = m2n × n2p × p2m = (m2 × m) × (n × n2) × (p × p2) = m3n3p3 

 for (iii) volume = 2q × 4q2 × 8q3 = 2 × 4 × 8 × q × q2 × q3 = 64q6 

EXERCISE 9.2 

1. Find the product of the following pairs of monomials. 

(i) 4, 7p

(ii) – 4p, 7p

(iii) – 4p, 7pq

(iv) 4p3, – 3p

(v) 4p, 0 

2. Find the areas of rectangles with the following pairs of monomials as their lengths and breadths respectively. 

(p, q); (10m, 5n); (20x2, 5y2); (4x, 3x2); (3mn, 4np) 

3. Complete the table of products. 

First monomial → Second monomial ↓ 

2x –5y 3x2 – 4xy 7x2y –9x2y2

4. Obtain the volume of rectangular boxes with the following length, breadth and height respectively. 

(i) 5a, 3a2, 7a4

(ii) 2p, 4q, 8r

(iii) xy, 2x2y, 2xy2

(iv) a, 2b, 3c

5. Obtain the product of 

(i) xy, yz, zx

(ii) a, – a2, a3

(iii) 2, 4y, 8y2, 16y3 

(iv) a, 2b, 3c, 6abc

(v) m, – mn, mnp

Example 5: Simplify the expressions and evaluate them as directed: TRY THESE 

Find the product: 

(4p2 + 5p + 7) × 3p

(i) x (x – 3) + 2 for x = 1,

(ii) 3y (2y – 7) – 3 (y – 4) – 63 for y = –2

Solution: 

(i) x (x – 3) + 2 = x2 – 3x + 2 

For x = 1, x2 – 3x + 2 = (1)2 – 3 (1) + 2 

= 1 – 3 + 2 = 3 – 3 = 0 

(ii) 3y (2y – 7) – 3 (y – 4) – 63 = 6y2 – 21y – 3y + 12 – 63 

= 6y2 – 24y – 51 

For y = –2, 6y2 – 24y – 51 = 6 (–2)2 – 24(–2) – 51 

= 6 × 4 + 24 × 2 – 51 

= 24 + 48 – 51 = 72 – 51 = 21 

Example 6: Add 

(i) 5m (3 – m) and 6m2 – 13m

(ii) 4y (3y2 + 5y – 7) and 2 (y3 – 4y2 + 5)

Solution: 

(i) First expression = 5m (3 – m) = (5m × 3) – (5m × m) = 15m – 5m2

Now adding the second expression to it,15m – 5m2 + 6m2 – 13m = m2 + 2m

(ii) The first expression

= 4y (3y2 + 5y – 7) = (4y × 3y2) + (4y × 5y) + (4y × (–7))

= 12y3 + 20y2 – 28y 

The second expression = 2 (y3 – 4y2 + 5) = 2y3 + 2 × (– 4y2) + 2 × 5 =2y3 – 8y2 + 10 

Adding the two expressions,

12y3 + 20y2 – 28y + 2y3 – 8y2 + 10 =14y3 + 12y2 – 28y + 10 

Example 7: Subtract 3pq (p – q) from 2pq (p + q). 

Solution: We have 3pq (p – q) = 3p2q – 3pq2 and 

 2pq (p + q) = 2p2q + 2pq2 

Subtracting,

2p2q + 2pq2 

3p2q – 3pq2 

– + 

– p2q + 5pq2

EXERCISE 9.3 

1. Carry out the multiplication of the expressions in each of the following pairs.

(i) 4p, q + r

(ii) ab, a – b

(iii) a + b, 7a2b2

(iv) a2 – 9, 4a

(v) pq + qr + rp, 0 

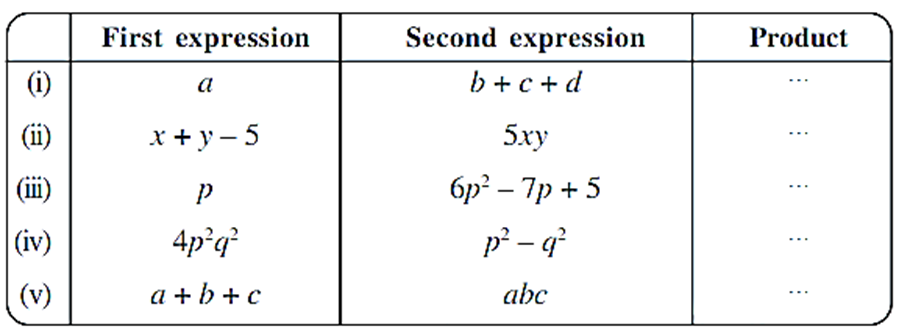
2. Complete the table. 

First expression Second expression Product 

(i) a b + c + d. . . 

(ii) x + y – 5 5xy . . . 

(iii) p 6p2 – 7p + 5 . . . 

(iv) 4p2q2 p2 – q2 . . . 

(v) a + b + c abc . . . 

3. Find the product.

4. (a) Simplify 3x (4x – 5) + 3 and find its values for

(i) x = 3

(ii) x = 1/2

(b) Simplify a (a2 + a + 1) + 5 and find its value for

(i) a = 0,

(ii) a = 1

(iii) a = – 1. 

5. (a) Add: p ( p – q), q ( q – r) and r ( r – p) 

(b) Add: 2x (z – x – y) and 2y (z – y – x) 

(c) Subtract: 3l (l – 4 m + 5 n) from 4l ( 10 n – 3 m + 2 l ) 

(d) Subtract: 3a (a + b + c ) – 2 b (a – b + c) from 4c ( – a + b + c )

Example 8: Multiply (i) (x – 4) and (2x + 3) (ii) (x – y) and (3x + 5y)

Solution: 

(i) (x – 4) × (2x + 3) = x × (2x + 3) – 4 × (2x + 3) 

= (x × 2x) + (x × 3) – (4 × 2x) – (4 × 3)

= 2x2 + 3x – 8x – 12 

= 2x2 – 5x – 12

(ii) (x – y) × (3x + 5y) = x × (3x + 5y) – y × (3x + 5y) 

= (x × 3x) + (x × 5y) – (y × 3x) – ( y × 5y) 

= 3x2 + 5xy – 3yx – 5y2 = 3x2 + 2xy – 5y2

Example 9: Multiply 

(i) (a + 7) and (b – 5) (ii) (a2 + 2b2) and (5a – 3b) 

Solution: 

(i) (a + 7) × (b – 5) = a × (b – 5) + 7 × (b – 5) 

 = ab – 5a + 7b – 35 

(ii) (a2 + 2b2) × (5a – 3b) = a2 (5a – 3b) + 2b2 × (5a – 3b) 

 = 5a3 – 3a2b + 10ab2 – 6b3

Example 10: Simplify (a + b) (2a – 3b + c) – (2a – 3b) c. 

Solution: (a + b) (2a – 3b + c)

= a (2a – 3b + c) + b (2a – 3b + c)

 = 2a2 – 3ab + ac + 2ab – 3b2 + bc 

= 2a2 – ab – 3b2 + bc + ac (Note, –3ab and 2ab are like terms) 

and (2a – 3b) c = 2ac – 3bc 

Therefore, 

(a + b) (2a – 3b + c) – (2a – 3b) c

= 2a2 – ab – 3b2 + bc + ac – (2ac – 3bc)

= 2a2 – ab – 3b2 + bc + ac – 2ac + 3bc 

= 2a2 – ab – 3b2 + (bc + 3bc) + (ac – 2ac) 

= 2a2 – 3b2 – ab + 4bc – ac

EXERCISE 9.4 

1. Multiply the binomials. (i) (2x + 5) and (4x – 3)

(ii) (y – 8) and (3y – 4) 

(iii) (2.5l – 0.5m) and (2.5l + 0.5m)

(iv) (a + 3b) and (x + 5) 

(v) (2pq + 3q2) and (3pq – 2q2) 

(vi)

 2. Find the product. 

(i) (5 – 2x) (3 + x)

(ii) (x + 7y) (7x – y) 

(iii) (a2 + b) (a + b2)

(iv) (p2 – q2) (2p + q) 

 

3. Simplify.(i) (x2 – 5) (x + 5) + 25

(ii) (a2 + 5) (b3 + 3) + 5 

(iii) (t + s2) (t2 – s) 

(iv) (a + b) (c – d) + (a – b) (c + d) + 2 (ac + bd) 

(v) (x + y)(2x + y) + (x + 2y)(x – y)

(vi) (x + y)(x2 – xy + y2) 

(vii) (1.5x – 4y)(1.5x + 4y + 3) – 4.5x + 12y 

(viii) (a + b + c)(a + b – c)

Example 11: Using the Identity (I), find (i) (2x + 3y)2(ii) 1032

Solution: 

(i) (2x + 3y)2 = (2x)2 + 2(2x) (3y) + (3y)2[Using the Identity (I)] 

= 4x2 + 12xy + 9y2 

(2x + 3y)2 = (2x + 3y) (2x + 3y) 

= (2x) (2x) + (2x) (3y) + (3y) (2x) + (3y) (3y) 

= 4x2 + 6xy + 6 yx + 9y2(as xy = yx) 

= 4x2 + 12xy + 9y2

(ii) (103)2 = (100 + 3)2 

= 1002 + 2 × 100 × 3 + 32(Using Identity I) 

= 10000 + 600 + 9 = 10609

Example 12: Using Identity (II),

find (i) (4p – 3q)2(ii) (4.9)2

Solution: 

(i) (4p – 3q)2 =(4p)2 – 2 (4p) (3q) + (3q)2[Using the Identity (II)] 

= 16p2 – 24pq + 9q2 

(ii) (4.9)2 =(5.0 – 0.1)2 = (5.0)2 – 2 (5.0) (0.1) + (0.1)2 

 = 25.00 – 1.00 + 0.01 = 24.01 

Example 13: Using Identity (III), find

(ii) 9832 – 172 = (983 + 17) (983 – 17) 

[Here a = 983, b =17, a2 – b2 = (a + b) (a – b)] Therefore, 9832 – 172 = 1000 × 966 = 966000

(iii) 194 × 206 = (200 – 6) × (200 + 6) = 2002 – 62 

= 40000 – 36 = 39964 

Example 14: Use the Identity (x + a) (x + b) = x2 + (a + b) x + ab to find the following: 

(i) 501 × 502 (ii) 95 × 103 

Solution: 

 (i) 501 × 502 = (500 + 1) × (500 + 2)

= 5002 + (1 + 2) × 500 + 1 × 2

= 250000 + 1500 + 2 = 251502 

 (ii) 95 × 103 = (100 – 5) × (100 + 3)

= 1002 + (–5 + 3) × 100 + (–5) × 3

= 10000 – 200 – 15 = 9785 

EXERCISE 9.5 

1. Use a suitable identity to get each of the following products. 

(i) (x + 3) (x + 3)

(ii) (2y + 5) (2y + 5)

(iii) (2a – 7) (2a – 7)

(iv) (3a – 1/2) (3a – 1/2)

(v) (1.1m – 0.4) (1.1m + 0.4) 

(vi) (a2 + b2) (– a2 + b2)

(vii) (6x – 7) (6x + 7)

(viii) (– a + c) (– a + c)

(ix)

2. Use the identity (x + a) (x + b) = x2 + (a + b) x + ab to find the following products. (i) (x + 3) (x + 7)

(ii) (4x + 5) (4x + 1)

(iii) (4x – 5) (4x – 1)

(iv) (4x + 5) (4x – 1)

(v) (2x + 5y) (2x + 3y)

(vi) (2a2 + 9) (2a2 + 5)

(vii) (xyz – 4) (xyz – 2) 

3. Find the following squares by using the identities. 

(i) (b – 7)2

(ii) (xy + 3z)2

(iii) (6x2 – 5y)2

(vi)

(v) (0.4p – 0.5q)2

(vi) (2xy + 5y)2 

4. Simplify.

(i) (a2 – b2)2

(ii) (2x + 5)2 – (2x – 5)2

(iii) (7m – 8n)2 + (7m + 8n)2

(iv) (4m + 5n)2 + (5m + 4n)2

(v) (2.5p – 1.5q)2 – (1.5p – 2.5q)2 

(vi) (ab + bc)2 – 2ab2c

(vii) (m2 – n2m)2 + 2m3n2

5. Show that. 

(i) (3x + 7)2 – 84x = (3x – 7)2

(ii) (9p – 5q)2 + 180pq = (9p + 5q)2

(iii) +

(iv) (4pq + 3q)2 – (4pq – 3q)2 = 48pq2 

(v) (a – b) (a + b) + (b – c) (b + c) + (c – a) (c + a) = 0

6. Using identities, evaluate. 

(i) 712

(ii) 992

(iii) 1022

(iv) 9982 

(v) 5.22

(vi) 297 × 303

(vii) 78 × 82

(viii) 8.92 

(ix) 10.5 × 9.5 

7. Using a2 – b2 = (a + b) (a – b), find 

(i) 512 – 492

(ii) (1.02)2 – (0.98)2

(iii) 1532 – 1472 

(iv) 12.12 – 7.92 

8. Using (x + a) (x + b) = x2 + (a + b) x + ab,

find (i) 103 × 104

(ii) 5.1 × 5.2

(iii) 103 × 98

(iv) 9.7 × 9.8