Class 07 ACTIVITY1 - LINES AND ANGLES

 ACTIVITY1 - LINES AND ANGLES

Objective: 

To verify that if two parallel lines are cut by a transversal, then each pair of corresponding angles are equal, by paper cutting and pasting.

Materials Required: 

White sheets of paper, colour pencils, geometry box, a pair of scissors, glue stick etc.

Procedure:

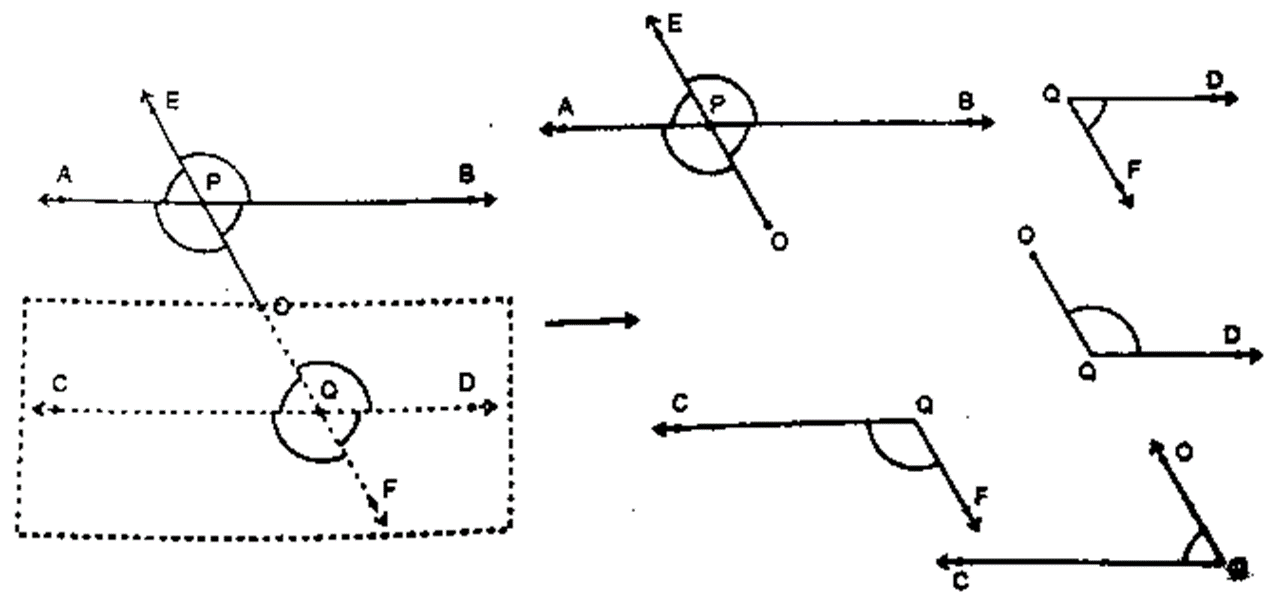
1. On a white sheet of paper, draw a pair of parallel lines AB and CD. Also, draw a transversal EF, cutting AB and CD at P and Q respectively. Mark the angles as shown in the figure. Mark a point O somewhere in the middle of PQ.

2. Cut the figure along the dotted lines to get four angular cut outs as shown below.

3. Paste the angular cutout ∠ DQO over ∠ BPE such that Q coincides with P and QD falls along PB.

4. Similarly, paste the angular cut outs ∠DQF,   ∠ CQF and ∠ CQO over ∠ BPO, ∠ APO and ∠ APE resp., such that in each case Q coincides with P and one arm of each angle falls along one arm of the corresponding angle.

Observations:

In figure1, AB ∥ CD and EF is Transversal. So, (∠ EPB, ∠ DQO), (∠ BPO, ∠ DQF), (∠ APE, ∠CQP) and (∠ APQ, ∠ CQF ) are four pairs of corresponding angles.

2. In figure, we see that if vertex Q of ∠ DQO coincides with the vertex P of ∠ BPE and QD falls along PB, then QO falls along PE, i.e., DQO completely overlaps BPE. So, ∠ DQO = ∠ BPE.

3. Similarly, in fig, we see that ∠ DQF, ∠ CQF and ∠ CQO completely overlap ∠ BPO, ∠ APO and ∠ APE resp.,

So, ∠ DQF = ∠ BPO, ∠ CQF = ∠ APO and ∠ CQO = ∠ APE

Conclusion : 

From the above activity, we can say that if two parallel lines are cut by a transversal, then each pair of corresponding angles are equal.

Do yourself: 

Verify the above property by drawing a pair of parallel lines which are 5 cm apart.